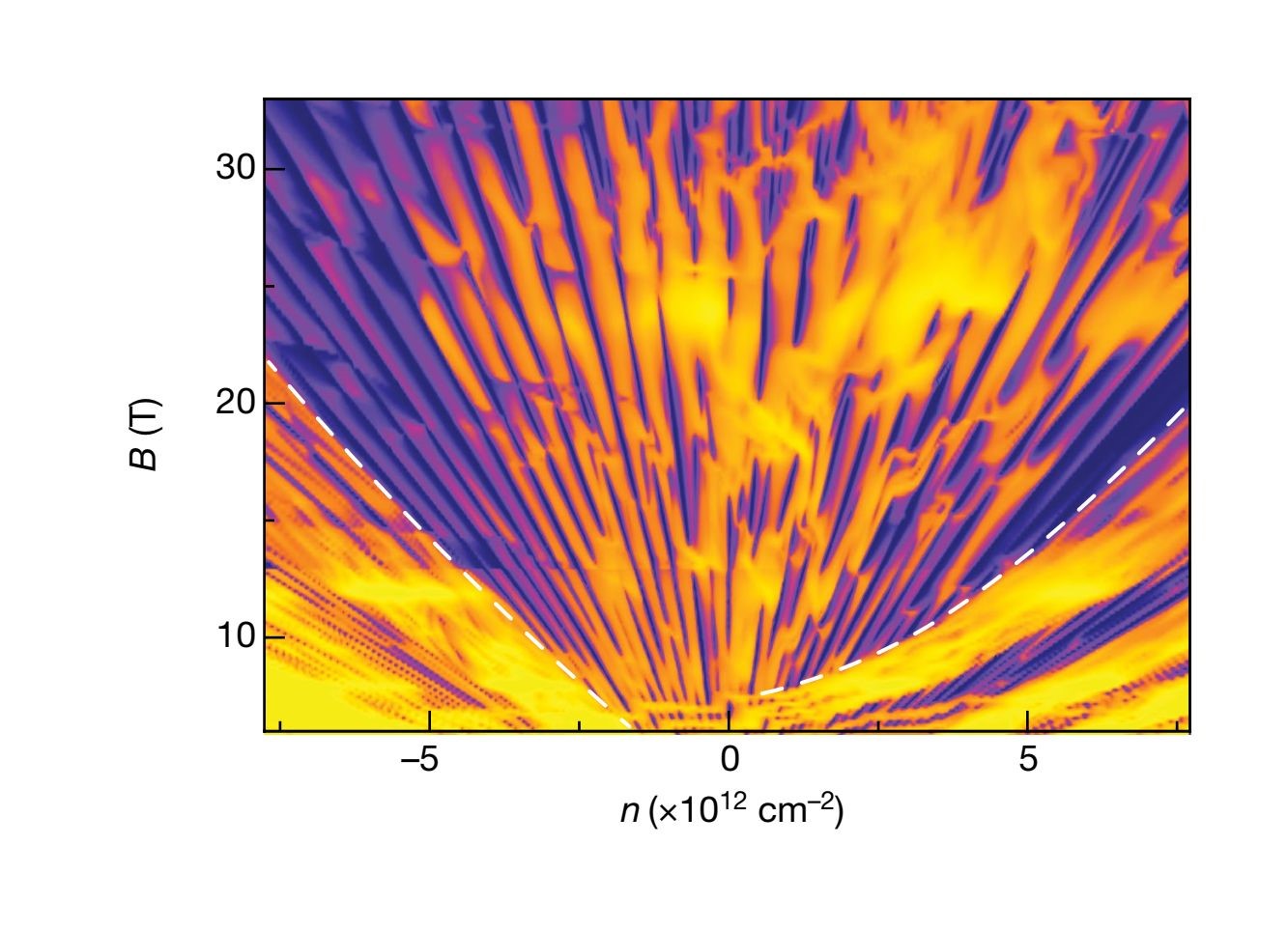
Graphite is made out of a stacking of layers of carbon atoms arranged in a honeycomb lattice. This recurring pattern gets disrupted at the surfaces of the crystal which leads to the occurrence of “surface states” – fading waves as one delves deeper into the bulk, which have been the subject of several investigations. In this work, “twistronics”, where one manipulates the properties of – usually two-dimensional (2D) – crystals through moiré patterns created by specific relative alignments between them, is taken one step further and applied to the surface of three dimensional graphite. More precisely, electrical transport in bulk Bernal-stacked graphite aligned with hexagonal boron nitride was studied under high magnetic fields, high enough to bring the magnetic length (giving the spatial extent of the electronic wave function) close to the moiré superlattice unit cell, as previously done to evidence the Hofstadter butterfly in graphene. Our findings revealed that the moiré pattern does not just alter the graphite surface states, it also has a significant impact on the electronic spectrum of the entire bulk of the graphite crystal. Drawing a parallel with the well-known fairy-tale of The Princess and The Pea, where the princess felt the pea right through the twenty mattresses and the twenty eiderdown duvets, the moiré influence extends from the surface all the way through graphite of over 40 atomic layers. As can be seen in the Figure, we observe a unique 2.5-dimensional (2.5D) intertwining of surface and bulk states that we describe as a 2.5D Hofstadter butterfly, a fractal version of the 2.5D quantum Hall effect discovered earlier on thin graphite, originating from the formation of confined vertical standing waves in the quantum limit. In the present case, the standing waves in graphite are orchestrated by twistronics, leading to the spiral dance of electrons trapped between the top and bottom surfaces to be directed both by the strong magnetic field and the moiré pattern. The extension of the surface moiré potential deep into the graphite bulk states opens a route to bring new non-trivial physics (spin-orbit coupling, ferromagnetism, and superconductivity) into graphite via proximity effects, and gives new prospects for controlling electronic properties in graphite or other semimetals.

Mixing of moiré-surface and bulk states in graphite, C. Mullan, S. Slizovskiy, J. Yin, Z. Wang, Q. Yang, S. Xu, Y. Yang, B. A. Piot, S. Hu, T. Taniguchi, K. Watanabe, K. S. Novoselov, A. K. Geim, V. I. Fal’ko, and A. Mishchenko, Nature 620, 756 (2023).

https://www.nature.com/articles/s41586-023-06264-5

Figure: 2.5-dimensional Hofstadter butterfly. Color map of graphite’s conductance (giving an image of the fractal electronic density of states) as a function of the magnetic field and the charge-carrier density. The white dashed curves indicate the transition from surface Landau levels to the bulk quantum regime.